|
2022年高考数学新高考Ⅰ-2<-->2022年高考数学新高考Ⅰ-4
(5分)在$\Delta ABC$中,点$D$在边$AB$上,$BD=2DA$.记$\overrightarrow{CA}=\overrightarrow{m}$,$\overrightarrow{CD}=\overrightarrow{n}$,则$\overrightarrow{CB}=($ )
A.$3\overrightarrow{m}-2\overrightarrow{n}$ B.$-2\overrightarrow{m}+3\overrightarrow{n}$ C.$3\overrightarrow{m}+2\overrightarrow{n}$ D.$2\overrightarrow{m}+3\overrightarrow{n}$
分析:直接利用平面向量的线性运算可得$\dfrac{1}{2}\overrightarrow{CB}=\dfrac{3}{2}\overrightarrow{CD}-\overrightarrow{CA}$,进而得解.
解:如图,
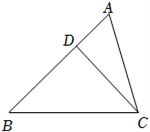
$\overrightarrow{CD}=\overrightarrow{CA}+\overrightarrow{AD}=\overrightarrow{CA}+\dfrac{1}{2}\overrightarrow{DB}=\overrightarrow{CA}+\dfrac{1}{2}(\overrightarrow{CB}-\overrightarrow{CD})=\overrightarrow{CA}+\dfrac{1}{2}\overrightarrow{CB}-\dfrac{1}{2}\overrightarrow{CD}$,
$\therefore$$\dfrac{1}{2}\overrightarrow{CB}=\dfrac{3}{2}\overrightarrow{CD}-\overrightarrow{CA}$,即$\overrightarrow{CB}=3\overrightarrow{CD}-2\overrightarrow{CA}=3\overrightarrow{n}-2\overrightarrow{m}$.
故选:$B$.
点评:本题主要考查平面向量的线性运算,考查运算求解能力,属于基础题.
2022年高考数学新高考Ⅰ-2<-->2022年高考数学新高考Ⅰ-4
全网搜索"2022年高考数学新高考Ⅰ-3"相关
|

