| 2023年高考数学新高考Ⅱ-20 |
|
2023-07-08 11:20:35 |
|
|
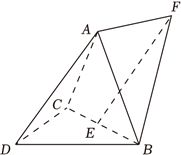
(12分)如图,三棱锥A−BCD中,DA=DB=DC,BD⊥CD,∠ADB=∠ADC=60∘,E为BC中点.
(1)证明BC⊥DA;
(2)点F满足→EF=→DA,求二面角D−AB−F的正弦值.
分析:(1)根据已知条件,推得DE⊥BC,AE⊥BC,再结合线面垂直的判定定理,即可求证.
(2)根据已知条件,推得AE⊥平面BCD,依次求出两个平面的法向量,再结合向量的夹角公式,即可求解.
证明:(1)连接AE,DE,
∵DB=DC,E为BC中点.
∴DE⊥BC,
又∵DA=DB=DC,∠ADB=∠ADC=60∘,
∴ΔACD与ΔABD 均为等边三角形,
∴AC=AB,
∴AE⊥BC,AE⋂DE=E,
∴BC⊥平面ADE,
∵AD⊂平面ADE,
∴BC⊥DA.
(2)解:设DA=DB=DC=2,
∴BC=2√2,
∵DE=AE=√2,AD=2,
∴AE2+DE2=4=AD2,
∴AE⊥DE,
又∵AE⊥BC,DE⋂BC=E,
∴AE⊥平面BCD,
以E为原点,建立如图所示空间直角坐标系,
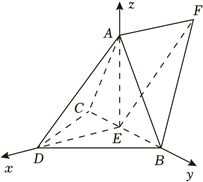
D(√2,0,0),A(0,0,√2),B(0,√2,0),E(0,0,0),
∵→EF=→DA,
∴F(−√2,0,√2),
∴→DA=(−√2,0,√2),→AB=(0,√2,−√2),→AF=(−√2,0,0),
设平面DAB与平面ABF的一个法向量分别为→n1=(x1,y1,z1),→n2=(x2,y2,z2),
则{−√2x1+√2z1=0√2y1−√2z1=0,令x1=1,解得y1=z1=1,
{√2y2−√2z2=0−√2x2=0,令y2=1,解得x2=0,z2=1,
故→n1=(1,1,1),→n2=(0,1,1),
设二面角D−AB−F的平面角为θ,
则|cosθ|=|→n1⋅→n2||→n1||→n2|=2√3×√2=√63,
故sinθ=√33,
所以二面角D−AB−F的正弦值为√33.
点评:本题主要考查二面角的平面角及其求法,考查转化能力,属于中档题.
|
|
http://x.91apu.com//shuxue/gkt/2023/2023xgk2/2023-07-08/33660.html |

